R
randy-gifford2
These have a very long build time. Cura slicer said the big size would take a couple of weeks to build and most of 1kg of filament to print. The little one would take 20 hours to build.
Useto navigate. Pressescto quit
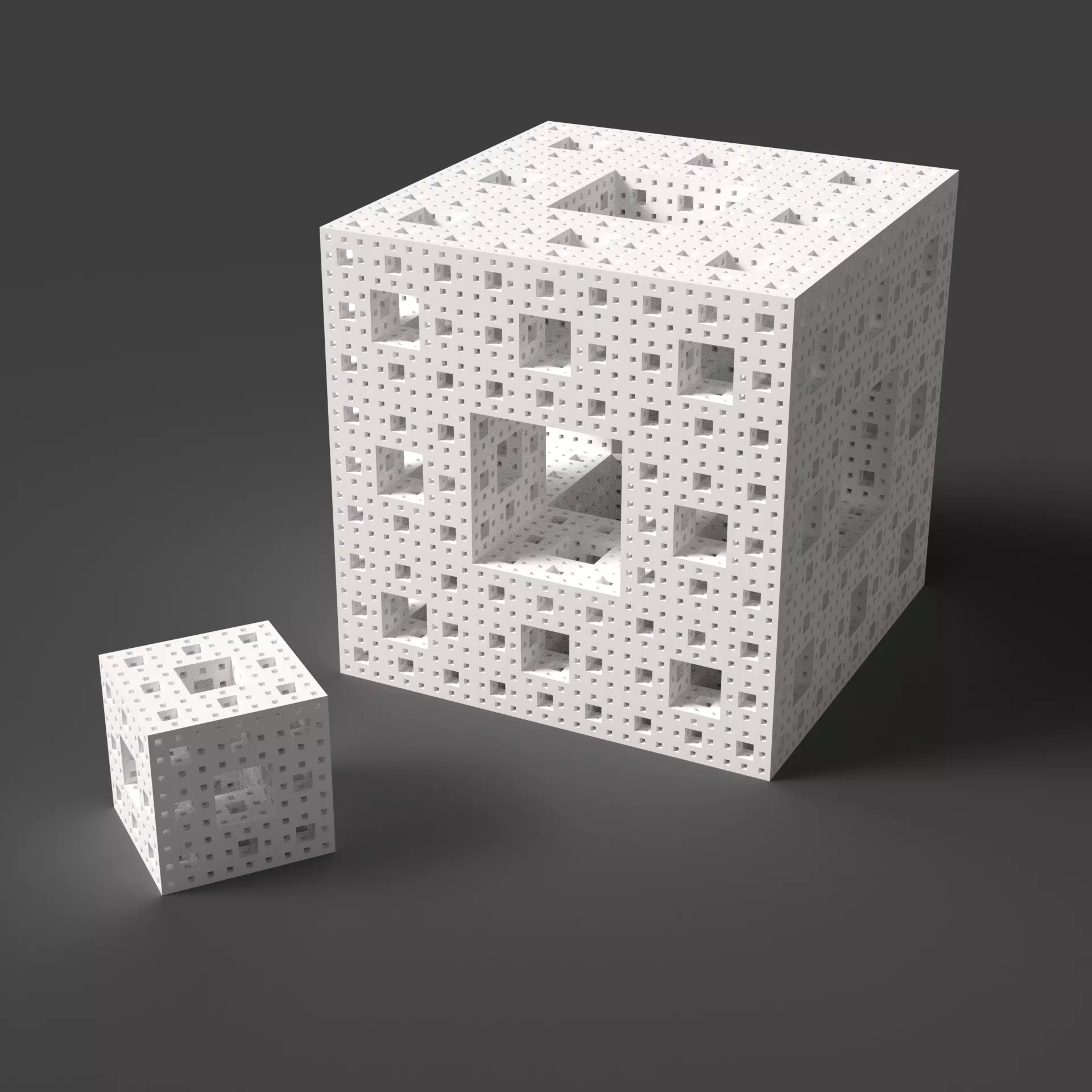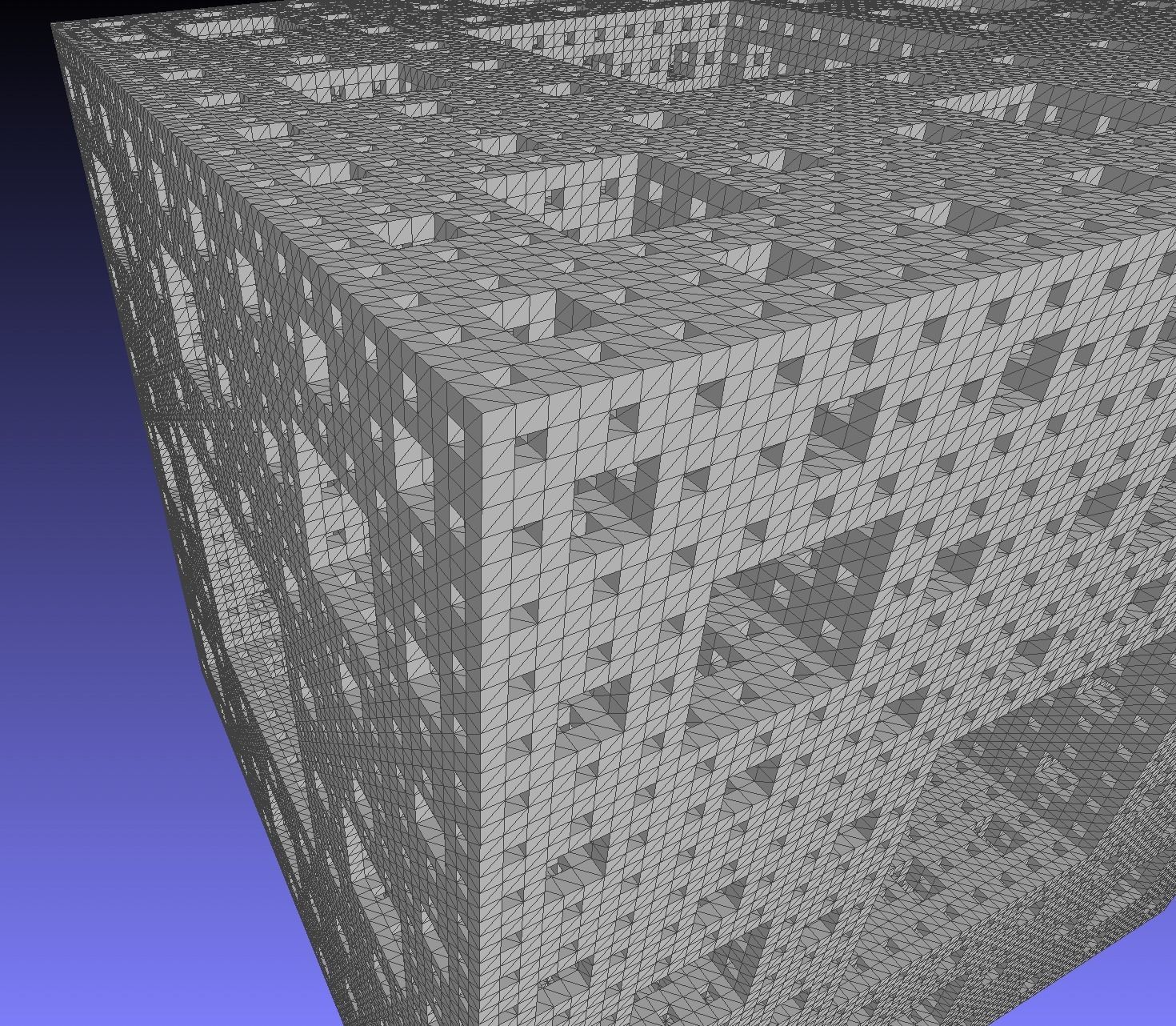
Menger Sponge Fractal Blocks 3D print model
Description
A pair of 3d-printable Menger Sponge fractal blocks.
Produced with a constructive solid geometry process.
The smaller block is ~36k polys, the larger block is ~673k polys.
The smaller block is proportionally smaller than the larger one as shown.
stl, obj, ply formats
The Menger sponge is a fascinating three-dimensional fractal shape that exemplifies the concept of self-similarity and is created through a recursive process of dividing and removing sections from a cube. While it may seem simple at its core, the Menger sponge becomes increasingly complex as you iterate this process, generating a structure with infinite surface area but zero volume.
3D printing a Menger sponge can be a challenging endeavor due to several reasons:
Intricate Structure: As the iterations increase, the Menger sponge's structure becomes incredibly intricate with many tiny details. This complexity poses challenges in terms of print resolution and precision.
Fragility: The smaller the iterations, the more fragile the resulting structure can become. Delicate features and thin walls can easily break during the printing process or when handling the finished object.
Support Material: Printing the Menger sponge often requires substantial support structures to prevent overhangs and ensure stability. Removing these supports without damaging the intricate structure can be labor-intensive.
Print Time: Creating a high-resolution Menger sponge with many iterations can be extremely time-consuming. The printer needs to navigate a large number of layers, slowing down the overall printing process.
Material Usage: Printing a Menger sponge with many iterations can consume a significant amount of material, especially if you're aiming for a large, detailed model.
Despite these challenges, 3D printing a Menger sponge can be a rewarding project for those interested in fractals and complex geometries. It serves as a great test of a 3D printer's capabilities and a demonstration of the fusion of mathematics and technology in the world of art and design.
Similar Models
to Menger Sponge Fractal Blocks
