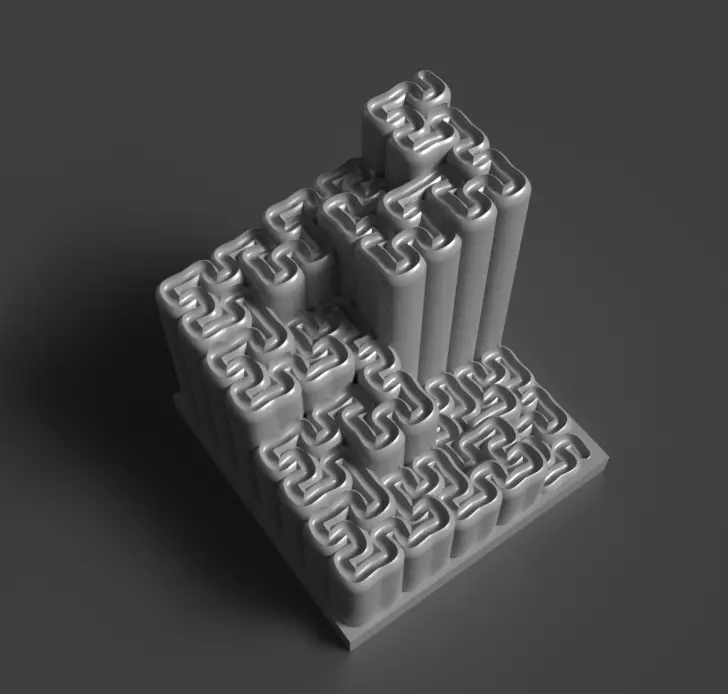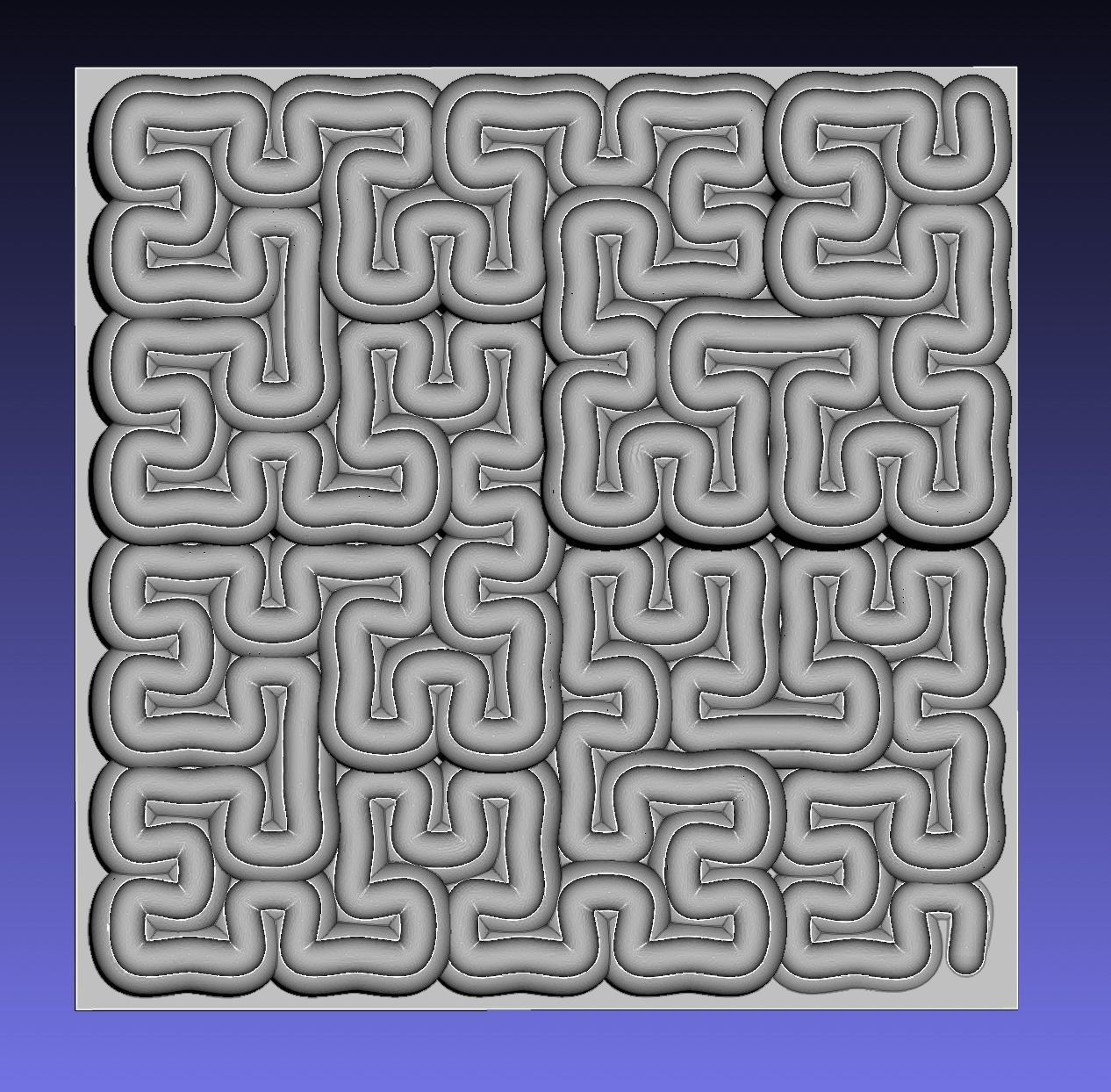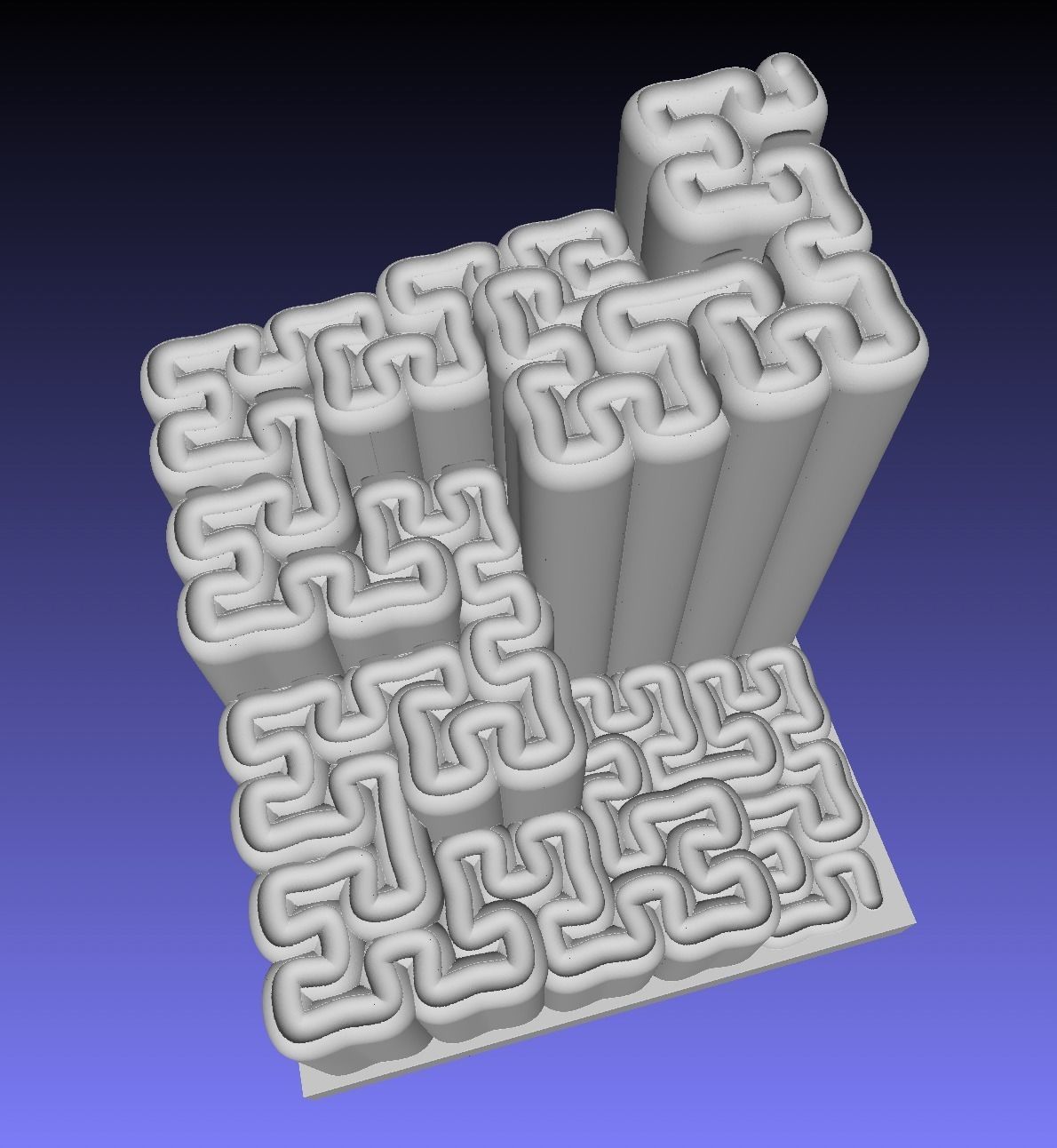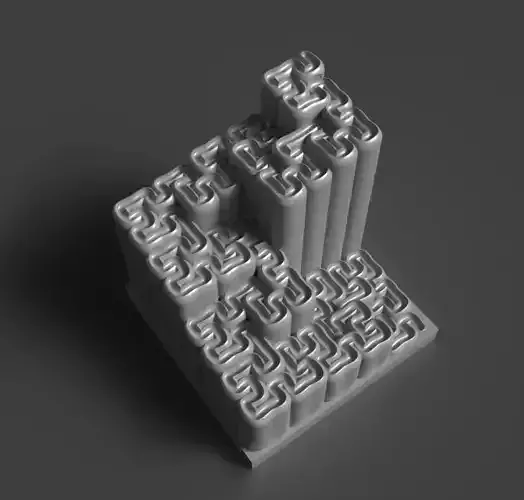
Hilbert Curve Fractal Marble Slide 3D print model

A 3d-printable fractal curve ramp based on a smoothed version of Hilbert's space-filling curve.

Created using a signed distance function / marching cubes technique followed by quadratic decimation.

1.6M polys

The Hilbert curve is a space-filling curve that systematically traverses a two-dimensional space in a way that maximizes locality, making it useful in various applications such as computer graphics, data indexing, and geographic information systems. It was introduced by German mathematician David Hilbert in 1891 as a way to map one-dimensional data onto a two-dimensional plane while preserving proximity relationships. The curve exhibits self-similarity and can be iteratively constructed, providing a mathematical framework for efficiently organizing and accessing data in multi-dimensional spaces.