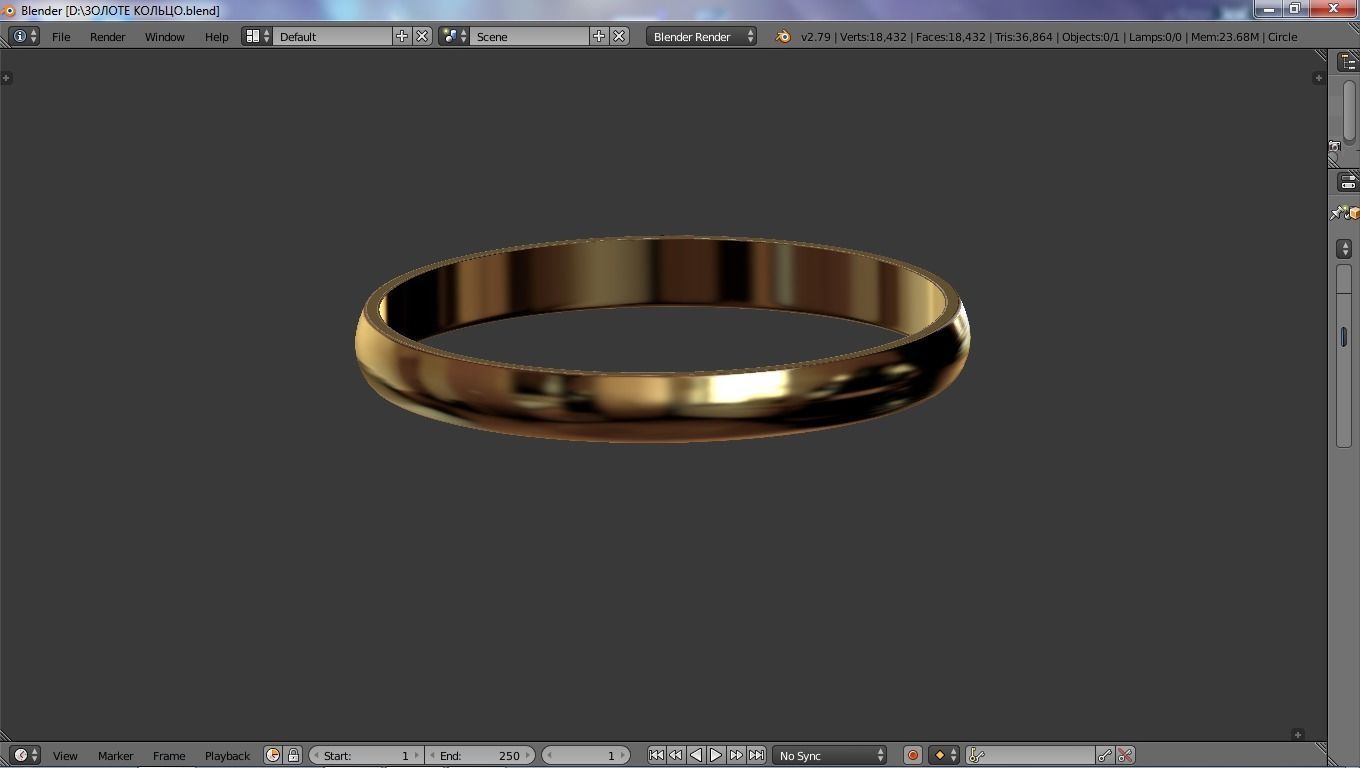
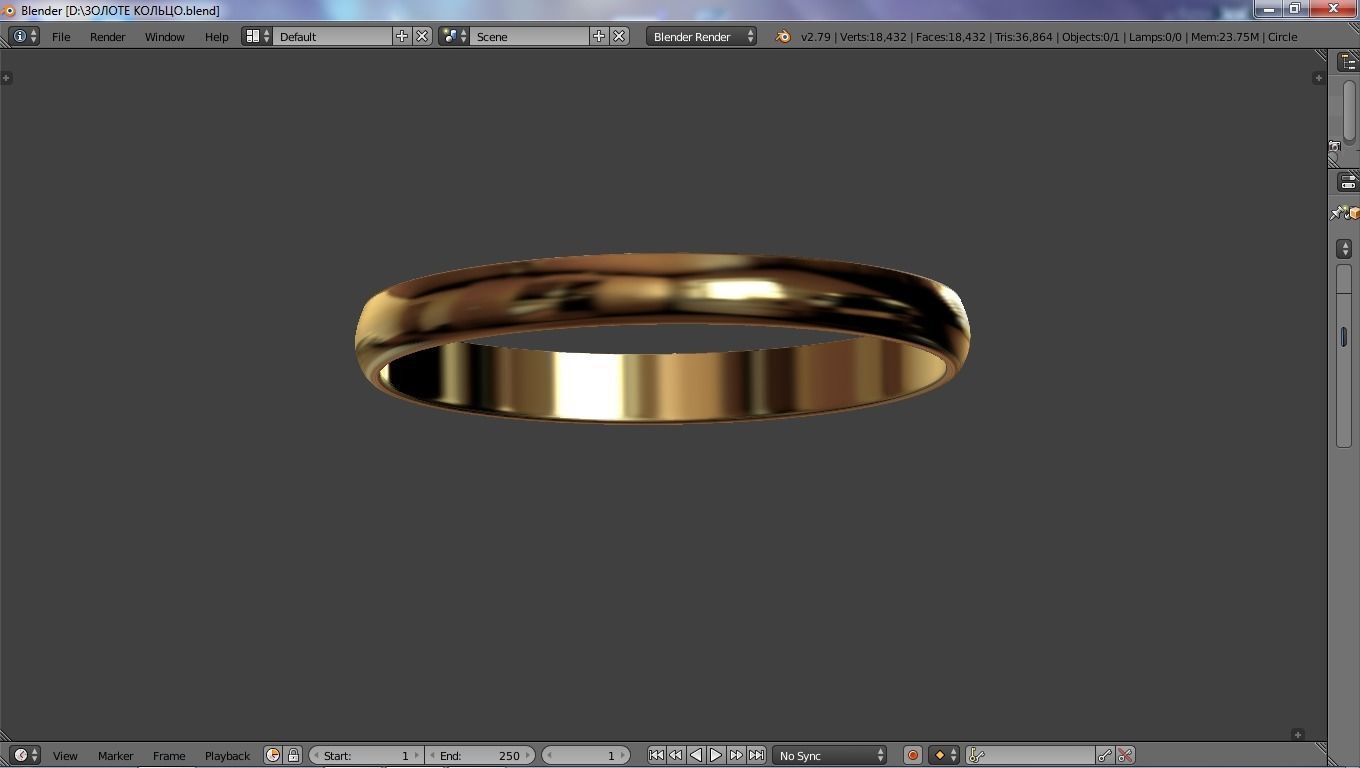
Gold ring 3D model

A ring (also an associative ring) in the general algebra is an algebraic structure in which the operation of reversible addition and the operation of multiplication, similar in properties to the corresponding operations on numbers, are defined. The simplest examples of rings are sets of numbers (integers, real, complex), a collection of numerical functions defined on a given set. In all cases there is a set that looks like a collection of numbers in the sense that its elements can be added and multiplied, and these operations behave naturally.To study the general properties of operations of multiplication and addition, their internal connection with each other, regardless of the nature of the elements over which operations are performed, and the concept of a ring.A ring (from an old Russian colo - a circle) [1] is a round object with an opening inside (example: torus or solid torus)

A ring (also an associative ring) in the general algebra is an algebraic structure in which the operation of reversible addition and the operation of multiplication, similar in properties to the corresponding operations on numbers, are defined. The simplest examples of rings are sets of numbers (integers, real, complex), a collection of numerical functions defined on a given set. In all cases there is a set that looks like a collection of numbers in the sense that its elements can be added and multiplied, and these operations behave naturally